On the proposed change of time marking to a decimal system: A plea that the duodecimal system be retained

by R. E. W. Goodridge
Honorary Treasurer of the Society

MHS Transactions, Series 1, No. 21
Read 25 February 1886

This article was published originally in MHS Transactions by the Manitoba Historical Society on the above date. We make this online version available as a free, public service. As an historical document, the article may contain language and views that are no longer in common use and may be culturally sensitive in nature. This online version was prepared using Optical Character Recognition software so that spelling and punctuation errors may have occurred inadvertently. If you find any such errors, please inform us, indicating the document name and error. Please direct all inquiries to webmaster@mhs.mb.ca. Help us keep history alive!

The wonderful improvements in locomotion and communication within the last few years have brought about a change in the marking the flight of time that would not have been expected by the past generation. Not very long ago it was seen that we had outgrown the idea of isolating each important town or city to keeping the time of its own meridian and we had the vast improvement introduced of dividing the circuit of the globe into twenty-four spaces of one hour each and keeping the same time over the whole of each of these spaces. The advantage of this change was that all over a large extent of country the clocks and watches kept the same time, and even between the dwellers on either side of the boundary lines there was always an agreement in the minute hands of the timepieces although the hour hands were not in accord. It was thenceforth an easy matter to compare the time between any two places on the globe. It was the travelers on the far-reaching longitudinal railways of this immense and progressive continent that were the first to perceive the advisability and to reap the benefit of the alteration, as by the new plan they had only to change the hour hand of the watch once perhaps in the course of a whole day's journey. We are now all accustomed to the so-called "Standard Time" and its easy adoption has emboldened a Professor London to propose a further change - also suggested by the experience of railway travelers. It has been noticed how great confusion is caused by the plan of having the hours of the day and night numbered with the same set of figures: 1 to 12, so that there is nothing to distinguish the record of the day from the night hours but the awkward insertion of the letters a.m. or p.m. to mark the difference, and the space for this is often grudged in the printing of the compact pages of the ordinary railway time tables.

To obviate this difficulty and also to assist even the stationary dwellers in the land, to whom also the hours of the day and night are nearly equally familiar, it has been suggested by the gentleman already named (Professor London) that we divide the day between one midnight and the next one into ten spaces only of 144 old minutes each, and each of these new hours into 100 new minutes (nearly half as long again as the old ones) and so on by hundreds or decimals until a small enough portion of time to suit all purposes is arrived at. This proposal is in accordance with the Decimal System, which is undeniably an excellent one, and which, as exemplified in the monetary system of Canada and the United States, is a most pleasant one to use for anyone who has been in the habit of working with the complicated money table of the old country. Intelligent people there have long been trying to introduce the decimal system, and it has often seemed strange that there is so great opposition to it, but now comes out a most important fact. The decimal system has its fault like most of us, and the great drawback to its adoption is that it is based upon a most awkward number or quantity and that is the number ten. Compared with twelve or one dozen, ten has no chance to please. You cannot divide ten by four or by three, but only by five or by two; whereas 12 is divisible by 6, 4, 3 and 2, and is just twice as convenient a number to work with. There is no other number like twelve for these advantages.

Think of the unsatisfying repeating figures constantly recurring in all decimal calculations, that would only occur half as often if we had 12 or one dozen as the base of our measurements which is the case in the duodecimal or dodecanal system, It is the masses of the people that cling to the convenience of the number twelve for use in the small transactions of their every day life, and to them how great is the help afforded in marketing when articles are sold by the dozen. They can divide that quantity so much better than they could ten to suit their wants. It is thus that in England the shilling of 12 pence is found so useful. For what a length of time has our notation been decimal and yet it is only comparatively in modern times that the decimal system has been introduced into tables of weights and measures. How difficult it is to get the people generally to make their purchases in quantities or measures of 100 and its divisions. And yet this is in the face of our notation having been decimal from the beginning. How different it would be if our notation were duodecimal. It is only the large transactions in business in modern times that are forcing us to shorten our labor in calculations by adopting tables in accordance with our notation. If the first reckoners had commenced with our present conveniences for writing and arithmetic instead of their ten fingers only, some better measure than ten would have been chosen. The evidence of practical arithmeticians now is that twelve is a better measure or radix than ten, but then comes in the fact of our having a decimal notation and it is thought to be easier to go on with the system we have than to make a change. Many may look upon our notation as a fixed institution like the climate or the law of gravity, and therefore to be accepted, and many fancy that in the decimal system they have attained the full height of excellence and do not look beyond it. The duodecimal system has been known by a few for a long time. It was taught in one of the principal military colleges in France about the beginning of the present century, but I have not met with record of its having been practiced elsewhere. It is thus referred to in the article on arithmetic in the "Encyclopedia Britannica:" "Ten is well chosen (as a base) being neither too large nor too small, but 12 might, in some respects, have been found more convenient. All numbers can be expressed with 12 or any other base, just as with 10." Ten does not even stand next to twelve as a measure to work with as sixteen has three measures - 2, 4 and 8, but sixteen has the same disadvantage as ten - namely, its indivisibility by 3.

Now look at our present way of measuring time. Is it not the most completely duodecimal of all our measures? We have twice twelve hours in the day, five times twelve minutes in the hour and the same number of seconds in the minute. We have twelve months in the year and the circuit of the heavens is divided into 12 signs of 30 degrees each or 30 times twelve degrees for the circle. When we know the superiority of the duodecimal measure it would be going backward instead of forward to introduce decimals into our time divisions, in which the better plan has prevailed from time immemorial Rather let us keep to our measure of 12 and divide the revolution of the globe from one midnight to the next into 12 spaces, either to be called hours, or, if preferred, by some new name, to show the difference from the old division. Then the clock faces would remain the same as before and the objection of having to speak of any hour above 12 would be avoided. It is rather curious, by the way, that such is apparently the strongest argument advanced against the proposed change from the present way of counting up to 12 twice in the same day, but it shows that in order to gain the public sympathy, any alteration proposed must have for its recommendation the greatest simplicity; and its terms and divisions be named by the shortest words.

We now come to this point, that having found a hindrance to a proposed improvement in time division or time marking, on account of the possibility of having to take to an inconvenient system, viz.: the decimal one, in order to simplify an old but cumbersome fashion, can we not find some better way?

One better way is certainly the duodecimal or dodecanal, but in order to reap the full benefit of it we would have to make a little change. The unanimity with which the adoption of Standard Time was so rapidly and thoroughly carried out is an evidence than the present age is advanced enough in intelligence to make an improvement the advantages of which are understood. There are always prejudices to be met with and overcome, especially in making a change from a custom that it has taken us some trouble to master. The spelling of the English language is an example of this. Difficulties have been left in the path of scholars, young and old, almost like the obstructions in a steeple chase, as if in order to test to the utmost the endurance of those engaged in the struggle. Long continuance in the use of clumsy implements (and words and figures cone under the category as well as spades and axes) is no reason why we should hesitate to change them for better, when we see them. We do our duty more thoroughly as pioneers in the many new and unexplored paths of science by clearing off the obstructions we meet with on our way instead of leaving them for others to stumble over as we may have done. We may save those who follow us from the chances of much valuable time being lost and possible disheartenments. Here is an opportunity to make calculations easier not only for ourselves but also for those who follow us in the faith that what has been good enough for their forerunners will do for them to begin with. We see the difficulties in the way but hitherto have not had the courage to clear them out of the path. We want two new figures for the numbers ten and eleven. Suppose we modify those in use and write X for ten and H for eleven. No fear of mistaking those marks for any of the other figures. Then we move the old written sign for ten (10) that used to be the completion of our measure, on to the new terminus twelve, which we now write 1Ø with some little mark, say a dot in the centre of the nought 0 or else over it to distinguish it from the old ten (10). We are now set up with all the figures necessary to carry out the duodecimal arithmetic. Next we require names for the new figures which is not quite so quickly done but is not impossible. This process will be rendered more simple if we first see if we cannot simplify the names we have so as to combine them the more easily with the new ones. Reference has already been made to the difficulties in spelling in our language. Let us take help from the advice of those who advocate phonetic reform, who often go back to the fountain head to get a pure and unadulterated word to take the place of something that has grown out of all shape and recognition. We can also choose short and euphonious words from other languages. We begin with

It will be observed that these names bear a strong resemblance to the names of the musical notes in Italian and there is a reason why they should do so. In the musical scale as it has been agreed on, and is in use among all civilized nations, there are just twelve recognized semi tones in the scale that has been (craving indulgence from musicians) clumsily condensed into an octave. Allow me here to ask if it is not possible for us to have a recognized name or number for each of those so-called semitones and raise each one of them to the dignity of having a place of its own on the staff. Give the staff six lines and do away with the marks for sharps or flats, always a trouble to anyone except the thoroughly expert. Abolish also the difference between the bass and treble staves which has been another hindrance to learners. Separate the staves on which the bass and treble have been hitherto written by the space of a complete octave, and then, for pianoforte music if you don't mind another little change, draw the lines up and down the page, or vertically instead of horizontally.

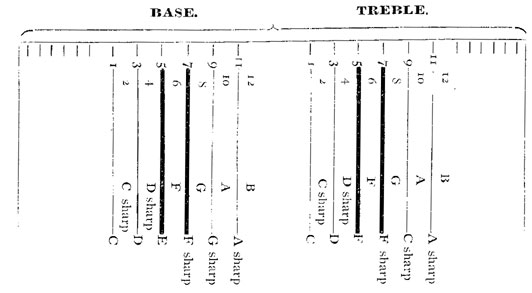
The above diagram is intended to show the proposed way of writing pianoforte or organ music, each group of six lines to contain a complete octave giving every semitone its own unchangeable position either on a line or in a space and doing away with marks for sharps or flats. It covers four octaves, sufficient for most ordinary compositions. The figures would not be required but a heavy mark should be placed opposite the line or space belonging to the key note of the composition. The music would be written as at present but progressing down the page instead of across. Words of vocal music could be printed in syllables down the centre space.

The stretch of an octave on the piano or organ is often too much for the hands of players. It would therefore not be out of place to propose to shorten it by taking one white key away and placing instead one black key. There would then be six white and six black keys arranged alternately, and they would correspond to the six lines and six spaces on the staff, requiring no effort on the part of the player to translate the position of the notes on the written music to the keyboard. The octaves could be marked off on the keyboard by coloring the first key of each group of six white ones. The playing of music on the piano or organ from notes would then be so easy as to shorten the time usually spent in learning it by quite one half. So much for the part that the number twelve already holds and may still further be yet developed to take in music.

This digression will have served its purpose if it has made us more tolerant of a change in our notation nomenclature. It is not given as the best possible but only as a suggestion as to how the desired end may be attained. We have got as far as the number twelve or do, the completion of one measure full. The next dozen is easy from the first, viz.:

I may here point out the foot of twelve inches as an illustration of the duodecimal system. It is a measure that has stood the test of the requirements of skilled artisans from all time and we do not hear of any change in it desired by them. We may take their acceptance of, and satisfaction with it, as the greatest possible evidence in its favor. Looking for a name for the cube of twelve, 1728 in our present notation or 1ØØØ in the duodecimal - we may try the name of cuba or cubo for that quantity. It is shorter than the word thousand, to which it would correspond in order, but of course represents a greater quantity. The next name wanted is that corresponding in order to our million and that (still looking to the cubic foot for illustration) being the cube of the inches in a cubic foot might be called cu-cuba or cu-cubo.

We have now got as far as is necessary in this rapid sketch of the duo-decimal system of arithmetic. I beg my hearers to bear in mind that this little paper is not written to advocate the immediate introduction of the number or quantity twelve into positions where it is not in use now but only for the sake of keeping it where it is already in use in order that it may be ready for us or our successors to be raised to its full dignity when its superiority over its rival ten shall have been fully recognized. We labor at present under the infliction of too many measures or bases in our tables of weights, measures and coinage. The numbers 7, 10, 12, 14, 16 and others are all mixed up in them, but I wish to point out that among them all the number twelve holds its own and takes its fair share of work, while ten is not even second to it in usefulness and favor except for the chance of its having the notation on its side. The decimal notation is a mistake and can be amended and the sooner the better. The best works will often bear revision and even re-revision.

If a change of the division of the day of 24 hours into a simpler measure is on the point of being actively advocated, or better still, has a chance of being carried out, let us hope that the new system we may obtain will leave us the old clock face with its twelve well balanced divisions to keep before us the most convenient quantity up till now in use, as the basis for the arithmetic of the future.

Page revised: 22 May 2010